A series of three interesting papers appeared today on astro-ph presenting a very exciting result: researchers at the Space Telescope Science Institute are finally able to predict with great precision the details of the collision of our Milky Way with the Andromeda galaxy, the nearest massive spiral galaxy.
Andromeda (M31) and the Milky Way are the two largest galaxies in the small group of galaxies called the Local Group. At the moment, M31 is about 770 kpc away from our galaxy, but Doppler measurements of the line-of-sight velocites of its stars have long shown that it is inexorably falling toward us. The exact nature of the collision, however, has so far remained unkwnon. Will it be a miss, glancing blow, or head-on smashup? This depends on the proper motion (sideways motion) of Andromeda in the sky, which is extremely hard to measure.
 The tangential motion of Andromeda has been sought for almost a century (see e.g. this paper, Barnard 1917), but very high astrometric precision is needed to obtain it. At last, the authors of this study manage to collect extraordinarily accurate Hubble Space Telescope observations of the tangential motion of M31 over a five-to seven-year period, and these data remove any doubt that Andromeda is destined to strike and merge with our Milky Way in four billion years. The Triangulum galaxy M33, the third most massive galaxy of the Local group, which is bound to M31, will likely join in the cosmic boom (with a small chance that M33 will hit the Milky Way first).
The tangential motion of Andromeda has been sought for almost a century (see e.g. this paper, Barnard 1917), but very high astrometric precision is needed to obtain it. At last, the authors of this study manage to collect extraordinarily accurate Hubble Space Telescope observations of the tangential motion of M31 over a five-to seven-year period, and these data remove any doubt that Andromeda is destined to strike and merge with our Milky Way in four billion years. The Triangulum galaxy M33, the third most massive galaxy of the Local group, which is bound to M31, will likely join in the cosmic boom (with a small chance that M33 will hit the Milky Way first).
Numerical simulations reproducing the observational data show that it will take an additional two billion years after the encounter for the interacting galaxies to completely plunge into each other. In this kind of merging event, stars inside each galaxy are so far apart that they do not collide. Instead, they will settle into different randomized orbits around the new galactic center to create an elliptical galaxy. According to the simulations, our Sun will be likely flung into a new, farther region of the galaxy, but neither the Solar System nor our Earth will be destroyed.
Paper I presents the observational proper motion data of M31. Papers II derives the velocity vector of M31 and uses it to constrain the mass of the Local Group. Finally, Paper III explores the future orbital evolution inside the Local Group by means of computer simulations. I will summarize each one of them in turn.
- Title: The M31 Velocity Vector. I. Hubble Space Telescope Proper Motion Measurements
- Authors: Sangmo Tony Sohn, Jay Anderson, Roeland P. van der Marel
- First Author’s Institution: Space Telescope Science Institute, Baltimore
The first paper of the series describes the challenging process of the derivation of proper motion measurement for M31. How challenging, you ask? Imagine that at the distance of Andromeda a transverse velocity of 100 km/s corresponds to a displacement in the sky as tiny as 0.027 mas/yr – only about ~20 cm/yr at the distance of the moon, ~20 meters/yr at the distance of the Sun. Indeed, even with very long time baselines (even greater than 40 years!) the accuracy of ground-based optical observations has not been enough to probe M31 transverse velocities. These first direct proper motion data for M31 are obtained with space-based observations of HST.
The onboard imagers of HST (e.g. ACS and WFC3) can achieve high accuracy by measuring the displacement of stars over time relative to background reference sources, objects that belong to the observed field and remain stationary over time due to their large distances. Quasi-stellar objects (QSOs) are typically adopted as reference sources because of their star-like appearance and high luminosity. However, the distribution of QSOs on the sky is pretty sparse and the field of view of HST imagers is pretty small, and this makes it quite difficult for the observer to find a QSO behind the field of interest. Even then, the uncertainty on proper motion measurements is limited by the positional accuracy of a single QSO. Therefore, instead of using QSOs as reference objects, the authors use background galaxies, which also look stationary at the spatial resolution of interest in this analysis. Even if galaxy positions are more difficult to measure than QSOs’, their much larger number provides a fundamental advantage in averaging.
The authors consider new V-band imaging HST data in three different fields in the Andromeda galaxy, one along the minor axis sampling primarily the M31 spheroid, one along the major axis sampling primarily the M31 outer disk, and a final field along M31’s Giant Southern Stream. They couple these recent data with pre-existing deep first-epoch observations, obtaining a 5-7 year time baselines. For each field, they measure the positions of thousands of M31 stars and hundreds of compact background galaxies. They build and fit a unique template for each individual object, in order to be able to recognize it in images taken at different epochs.
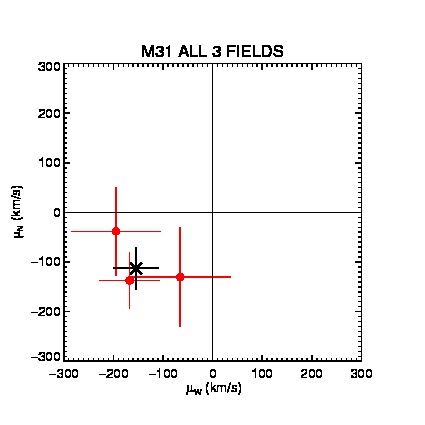
Figure 1. Average proper motions (km/s) of M31 in the three fields (red circles). The error-weighted mean of the 3 fields is shown as the black X mark.
Taking the difference between the second- and first-epoch positions of the galaxies, they obtain the relative displacement of the galaxies with respect to the M31 stars. Since in reality the galaxies are stationary and the stars are moving, what they really get is the displacement of the M31 stars, which can be immediately turned into proper motion via division by the time baseline.
Knowing the distance of the Andromeda galaxy, the average stellar proper motions for each field can be converted in velocities in the North and West direction, as shown in Figure 1, together with their global average over the three fields.
The final accuracy of the 5-7 year time baseline observations is only 12 μas/yr, and the robustness of the measurements are supported by the fact that data from different instruments, taken at different times and with different telescope orientations, as well as measurements of different fields, all yield statistically consistent results.
The current data could be improved by future additional HST observations. Also, soon it will be possible to compare them with independent water maser measurements for individual sources in M31 (see this Letter), which might allow measurement of other cool effects, such as the M31 proper motion rotation, and the increase in Andromeda’s apparent size due to its motion towards us.
The following video from the NASA press release zooms into the neighboring Andromeda galaxy, showing the images of the same halo field taken seven years apart and used to measure the proper motion relative to background galaxies. When the shift on the sky is projected over the next 32,000 years, the lateral motion becomes apparent.
- Title: The M31 Velocity Vector. II. Radial Orbit Towards the Milky Way and Implied Local Group Mass
- Authors: Roeland P. van der Marel, Mark Fardal, Gurtina Besla, Rachael L. Beaton, Sangmo Tony Sohn, Jay Anderson, Tom Brown, Puragra Guhathakurta
- First Author’s Institution: Space Telescope Science Institute, Baltimore
In the second paper, the HST proper motions of M31 presented in Paper I are used to determine first the direction and speed of Andromeda – its velocity vector – with respect to the Milky Way, and then the mass of the Local Group.
In order to derive the velocity vector of M31, the measurements of stellar proper motions have to be corrected for the contributions from the motion of the center-of-mass of M31 and for the stellar motions internal to the Andromeda Galaxy. This kind of corrections are complicated by the fact that in each field observed with HST, different fractions of the stars are contributed by different structural components of the Andromeda Galaxy, namely disk, bulge, halo, or material currently being accreted. To estimate the center-of-mass motion, one needs to know for each field both the fraction of the stars in each component, and the transverse kinematics of those stars, which are not constrained observationally.
The easiest way to tackle the problem is via a dynamical N-body model of the mass and light in M31. Thus, based on their previous works on the topic, the authors build a particle model which contains a bulge, a disk, and a halo (dark matter plus stars), and which reproduces the surface-brightness profiles of the different components reasonably well, and also satisfies a series of kinematic constraints, including the disk rotation curve, the bulge velocity dispersion, and constraints on the halo mass from statistical tracers such as globular clusters, planetary nebulae, satellite galaxies, and red giant stars. In a fixed potential corresponding to this particle model, the Giant Southern Stream of the Andromeda Galaxy is created by simulating the disruption of a satellite galaxy.
The final N-body model resembling the detailed structure of M31 is used to estimate the fractional contributions of the stars belonging to different structural components, and the center-of-mass motion. The corrections for internal kinematics make only a small difference (less than 10 km/s) for the final transverse velocity estimate, which is consistent with independent estimates using the kinematics of satellite galaxies of M31 and the Local Group.
The proper motion of M31 measured in Paper I includes the Sun’s motion about the Galaxy, which needs to be subtracted off to get the true transverse velocity with respect to the Milky Way. This additional correction can be done using the most recent estimates of the solar motion within the Milky Way. The final result is that M31 has a radial velocity with respect to the Milky Way of about -109 km/s, and a tangential velocity of about 17.0 km/s (<34.3 km/s at 1-sigma confidence). These numbers clearly imply that the velocity vector of Andromeda Galaxy is statistically consistent with a radial (head-on collision) orbit towards the Milky Way. This means that the Milky Way-M31 system is bound, and that the two galaxies will merge, as investigated further in Paper III.
As an additional bonus, the velocity vector of M31 is used to further refine the mass estimate of the Local Group, applying the so-called Local Group timing argument. A Bayesian combination (see this astrobite) with other mass estimates yields a total mass of the Local group of about 3 times 1012 solar masses.
Finally, the authors present a range of arguments, based on the masses and relative motions of M31 and M33 (whose transverse motion is measured from water-maser studies), indicating that the Andromeda Galaxy and the Triagulum Galaxy are actually a bound pair, in agrement with observational evidences of tidal deformation between M33 and M31.
- Title: The M31 Velocity Vector. III. Future Milky Way-M31-M33 Orbital Evolution, Merging, and Fate of the Sun
- Authors: Roeland P. van der Marel, Gurtina Besla, T. J. Cox, Sangmo Tony Sohn and Jay Anderson
- First Author’s Institution: Space Telescope Science Institute, Baltimore
The final paper of this amazing series investigates the future dynamical evolution of the Milky Way-M31-M33 system with two complementary methods: N-body simulations and semi-analytic orbit integrations.
The N-body simulations are performed with the N-body smoothed particle hydrodynamics code GADGET-3, and allow the authors to study the evolution of each galaxy in great detail. However, they are computationally expensive, and therefore only a limited number of possible initial conditions can be explored with this approach. In contrast, the faster, semi-analytic orbit integration methods permit them to explore the whole parameter space of initial conditions allowed by the observational uncertainties.
The authors study the orbital evolution of several models, exploiting Monte-Carlo techniques, and these allow them to get an “average” assessment of what will happen to the Milky Way, the Andromeda galaxy, and even our Sun in the far future.Their numerical simulations start at the present epoch, with initial positions and orientations reproducing the current conditions. This means that, for the first time, the initial conditions of these simulations are fully observationally constrained. In particular, the Galactocentric tangential velocity of M31, inferred in Papers I and II, puts significant constraints on the future orbital evolution and merging of the system, compared to what was known so far.
The simulations show that the Milky Way and the Andromeda galaxy will merge, consistently with the radial (head-on collision) orbit deduced in Paper II for M31, and that the first “pericenter” (closest approach) will occur at around 4 Gyr from now. For our Milky Way, the encounter has 72.2% probability of being prograde (the galaxy spins in the same direction as the flyby). In 41.0% of the Monte-Carlo orbits M31 makes a direct hit with our Milky Way, where the authors define a “direct hit” as an encounter with a first pericenter distance less than 25 kpc. The two galaxies will eventually merge after 5.9 Gyr, and the radial mass profile of the merger remnant will be significantly more extended than the original individual profiles. Roughly speaking, this profile will follow the R1/4 law characteristic of elliptical galaxies, in agreement with the predictions from the numerical simulations of major mergers of spiral galaxies.
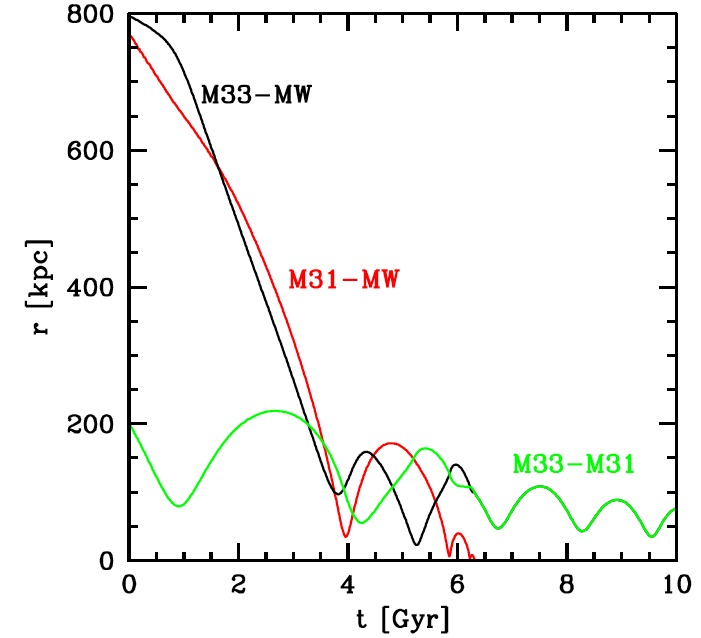
The separation between the galaxies in the Milky Way-M31-M33 system as a function of time, calculated with N-body simulations for the "canonical" model of Paper III, i.e. the model taking the new M31 transverse motion into account.
Do not forget that as M31 approaches our Galaxy, M33 is orbiting around it. For the pair M31-M33, the simulations predict that the first closest approach will happen at less than 1 Gyr from now. By the time M31 gets to its first closest approach with the Milky Way, M33 is close to its second pericenter with M31. The Triangulum galaxy (M33) can have several different types of orbits with respect to the merging pair of the Milky Way and the Andromeda galaxies. In 9.3% of the Monte-Carlo orbits, M33 makes a direct hit with the Milky Way at its first pericenter, 3.7 Gyr from now, even before M31 reaches pericenter or collides with our Galaxy. In 7.2% of the Monte-Carlo orbits, M33 gets even ejected from the Local Group. Then, it either falls back and merges with the elliptical merger remnant much later, or it becomes completely unbound from the system. In the remaining (most) 83.5% of Monte-Carlo orbits, M33 does not make a direct hit with the Milky Way on its first pericenter, and it does not leave the Local Group. In these orbits, which are the majority, the Milky Way and Andromeda galaxy merge first, with M33 orbiting around them, and perhaps joining in later.
Finally, by identifying candidate suns in their N-body models, the authors are able to predict what will happen to our Sun during the evolution of the Milky Way-M31-M33 system. In galaxy collisions, individual stars essentially never collide, because they are so far apart compared to their size – it’s the gas and dust that interacts. Simulations predict that there is a chance that the Sun ends up near the center of the merger remnant, but much more likely (85.4% probability) it will end up at larger distances than the current ones from the galactic center. There is a 20.1% probability that the Sun will at some time in the next 10 Gyr find itself moving within 10 kpc of M33, but still remain dynamically bound to the Milky Way-Andromeda merger remnant. 
Of course, the environment of the Sun and the solar system will be affected by all these strikes and passages, and a consequent change in their evolution is not unlikely. For instance, the structure of the outer solar system can be altered by nearby passages of other stars.
If a very close passage were to affect the Earth orbit and relocate our planet in the solar system relative to the habitable zone, it could even affect life (several billion years from now).
The huge collision of M31 (and possibly M33) with the Milky Way in 4 billion years from now is the next major (and predictable) cosmic event affecting the environment of our Sun and solar system. The exhaustion of the Sun’s core of hydrogen fuel will happen about 2.5 Gyr later, but, as the Sun’s luminosity slowly increases over time, changes in the Earth’s temperature and climate may well make life on Earth impossible well ahead.
To conclude, here is a great video, again associated to the NASA press release, showing a nighttime sky view of the future encounter between our Milky Way and the Andromeda galaxy, ultimately creating a huge elliptical galaxy. In particular, note how, as M31 approaches the Milky Way, the latter begins to show distortion due to tidal pull from Andromeda, and how, during the first close approach (after 3.8-3.9 billion years), the sky shines with new star formation. The view of the titanic collision from our solar system will most likely be different, depending on how the Sun’s orbit within the galaxy changes during the strike.



Trackbacks/Pingbacks