Title: Binary Evolution Leads to Two Populations of White Dwarf Companions
Authors: David S. Spiegal
First Author’s Institution: Institute of Advanced Study,  Princeton, NJ 08540

Imagine you are a planet or a brown dwarf orbiting around a low mass star (i.e., a star with a mass of 1-3 solar masses) and then all of a sudden your host star evolves off the main sequence (MS). Once the star leaves the  MS, its core will contract and its envelope will expand causing the star to become a subgiant or giant star. As you watch your host star begin to expand you begin to ask yourself “What’s going to happen to me? Will I be engulfed by the star? Pushed outwards or inwards? Completely unaffected?” It turns out the answer to this question depends on the distance you orbit your host star, the star’s inertia and mass-loss as it evolves off the MS, the tidal torques conveyed between you and your host star, and the total angular momentum of the binary system.

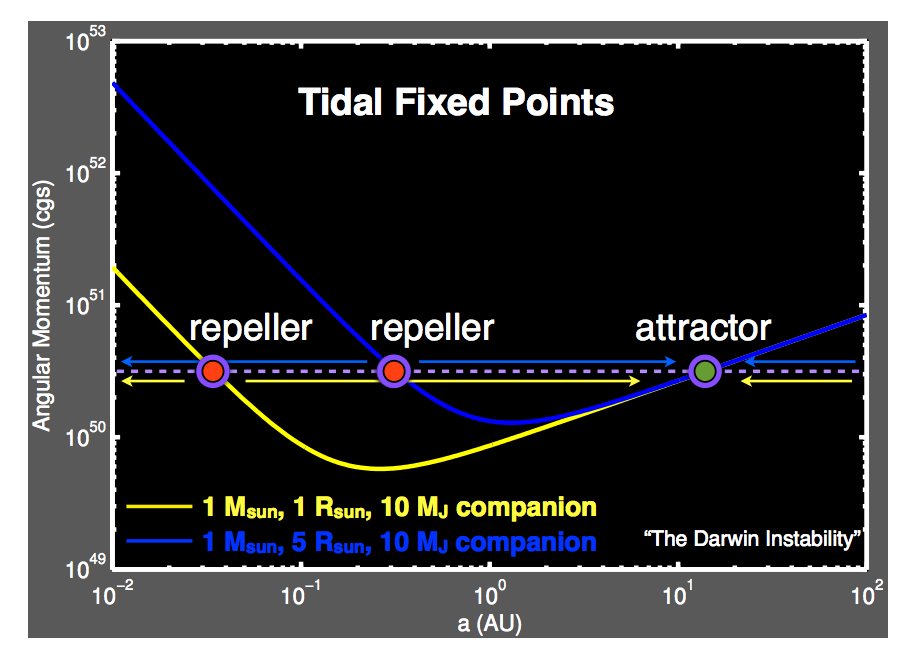
The curves represent the orbital radius/angluar momentum pairs for a binary system. The x-axis represents the average separation (semi-major axis) between the low-mass companion and star in units of AU (note 1 AU = distance between the Sun and Earth). The yellow curve shows this relationship for a sunlike MS star with a Jupitar mass companion whereas the blue curve represents the same binary after the star has evolved off the main sequence and has expanded to 5 solar radii. The stellar radius only affects the repeller point!

In this article, the author considers the ultimate fate of the low-mass companions of a star that evolves off the main sequence and will eventually become a white dwarf. The post-MS evolution of the host star can rapidly change the dynamical evolution of the system. For example, mass loss from the star will cause the companion to move outwards whereas tidal torques conveyed between the star and its companion will cause the companion to move inwards. As the star expands its moment of inertia will also change and effect the dynamical evolution.

As the system evolves it will try to reach equilibrium and become tidally locked. In this state, the net torque on the system vanishes and for any total angular momentum of the system there exists two orbital radii for which this state occurs as shown in the figure below. The inner location (the repeller) turns out to be unstable. In other words, if the low-mass companion is located within this orbital radius it will plunge inwards and merge with the star. On the other hand,  if it is located outside of the inner stability point it will move towards the outer stability point (the attractor). The figure above shows that as the star expands the repeller point moves outwards but the attractor point remains unchanged. Thus, if the dynamical evolution is unable to move the companion outwards quickly enough then the planet will be engulfed by the star and enter the common envelope (CE) phase.

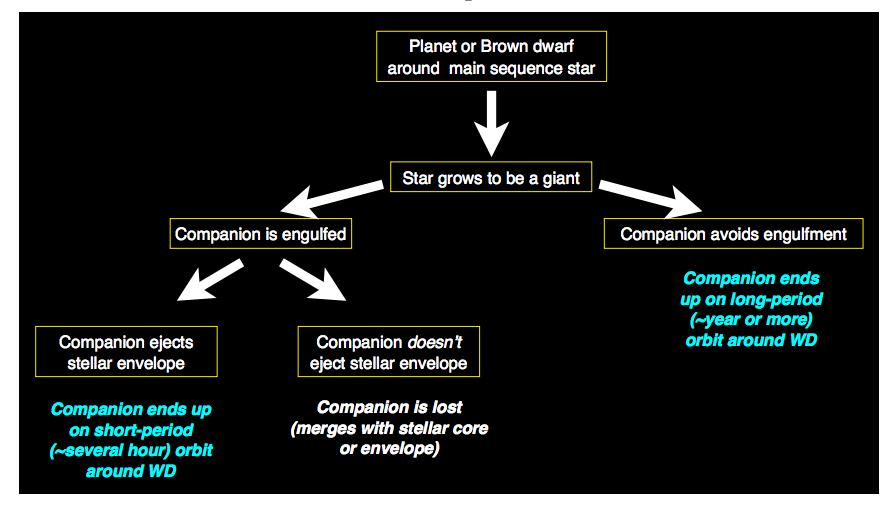
Summary of the possible evolutionary paths that a binary system can follow. The three types of outcomes are: (1) the companion can avoid being engulfed by the primary and end up far away from the white dwarf on a long-period orbit or a companion can be swallowed by the evolving star and can either (2) survive the CE phase and end up in a very close-in, short period orbit around the white dwarf or (3) be destroyed during the CE phase.

During the common envelope phase the engulfed companion will spiral inwards. If the companion is massive enough then it will sink through the stellar envelope (i.e., the star’s outer layers) without being tidally disrupted and the orbital energy of the binary system will decrease. If this decrease in orbital energy exceeds the gravitational binding energy of the stellar envelope, then the stellar envelope will be ejected (assuming the lost orbital energy goes into unbinding the stellar envelope). This leads to two possible scenarios if the CE phase occurs: (1) the companion will end up very close to the host star if the envelope is ejected or (2) the companion will merge with the star’s core or become tidally shredded if the envelope is not ejected. The figure below shows the allowed orbital radii of companions that survive the common envelope phase.

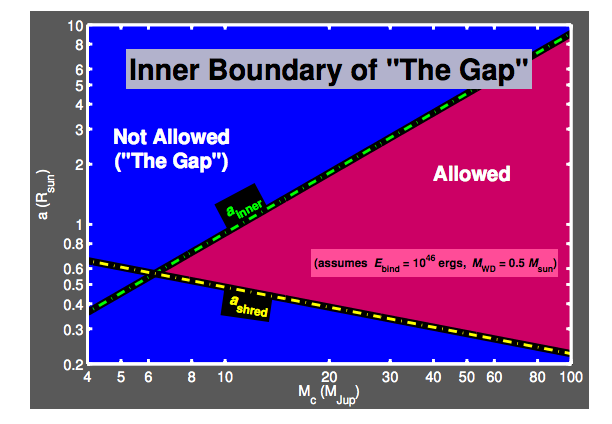
Interior to the period/orbital-radius gap for binary companions to white dwarfs. The y-axis represents the final separation (semi-major axis) between the low-mass companion and star in un its of the solar radius. The magenta region shows the allowed orbital radii of low-mass companions that survive the CE envelope phase by ejection of the envelope before the companion is either tidally shredded or merges with the stellar core.

In summary, the author finds that the post-MS evolution can significantly affect the orbital radii of low-mass binary companions around the resulting white dwarf. Companions which are too close will be swallowed by the expanding star. Once the companion is consumed it will either be tidally shredded if it’s mass is low enough, merge with the core if the decrease in orbital energy is not large enough to unbind the envelope, or obtain an extremely close in orbital radius (<1 solar radius) if the envelope is ejected during the CE phase. If the companion avoids engulfment by the expanding star then it will continue to move outwards due to mass loss from the host star.