English
Title: An Independent Analysis of the Six Recently Claimed Exomoon Candidates
Authors: David Kipping
First Author’s Institution: Department of Astronomy, Columbia University, New York, NY, USA
Status: Accepted to ApJ Letters [closed access]
Moons in our Solar System are very common. In fact, we see one every night. We also know about Phobos and Deimos, the ocean worlds of Jupiter, and even about methane on the largest of Saturn’s moons. The idea of a planet having a moon is so natural for us – we’ve observed tons of them in the Solar System. However, do planets in other planetary systems (we call them ‘exoplanets’) have moons? Well, we don’t know if we’ve observed any yet. In the literature, there is only one claimed “exomoon” (moon of a planet in a planetary system beyond ours). A recent study claims to have found six new exomoons. This is a big deal. If validated, such a discovery would represent a giant leap forward in the detection of exomoons. In our Solar System, studies of the moons have been able to tell us a lot about the formation of the system, the composition of the planets, and potential clues to how life began on Earth. Therefore, the discoveries of exomoons could help us understand the exoplanets we are observing. Today’s paper made a rigorous analysis of each of these six exomoon candidates and did not conclude any of these candidates to be an exomoon.
Six Keplerian Objects of Interest (KOI) are proposed to host exomoon candidates: KOIs-268.01, 303.01, 1888.01, 1925.01, 2728.01, and 3320.01. Finding an exoplanet is a challenging problem, but finding exomoons is even more challenging. Despite the great success of searches for exoplanets, exomoons are difficult to detect by all existing methods of such searches since they need to be of a significant size to get noticed. That is why different statistical analyses are done to find exomoons around detected exoplanets. The analysis in this paper is based on three questions. According to David Kipping, for a real exomoon, the answers to all these questions should be yes.
Question 1: Do we see a wobbling of the planets?
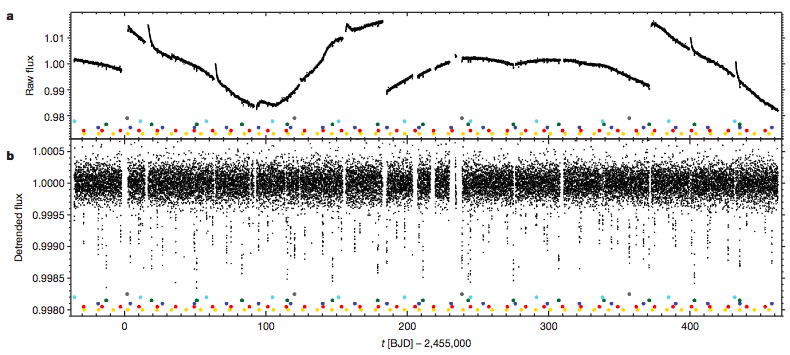
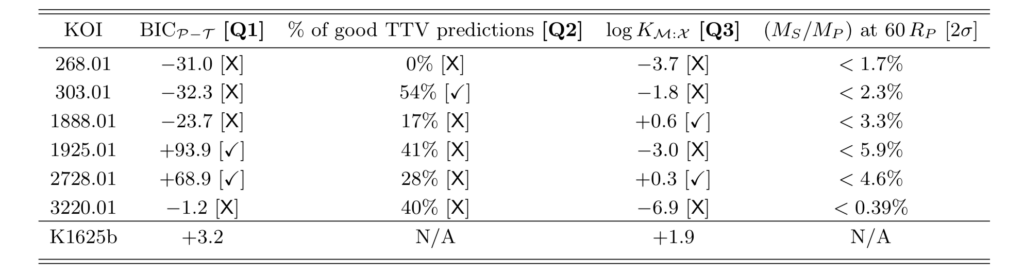
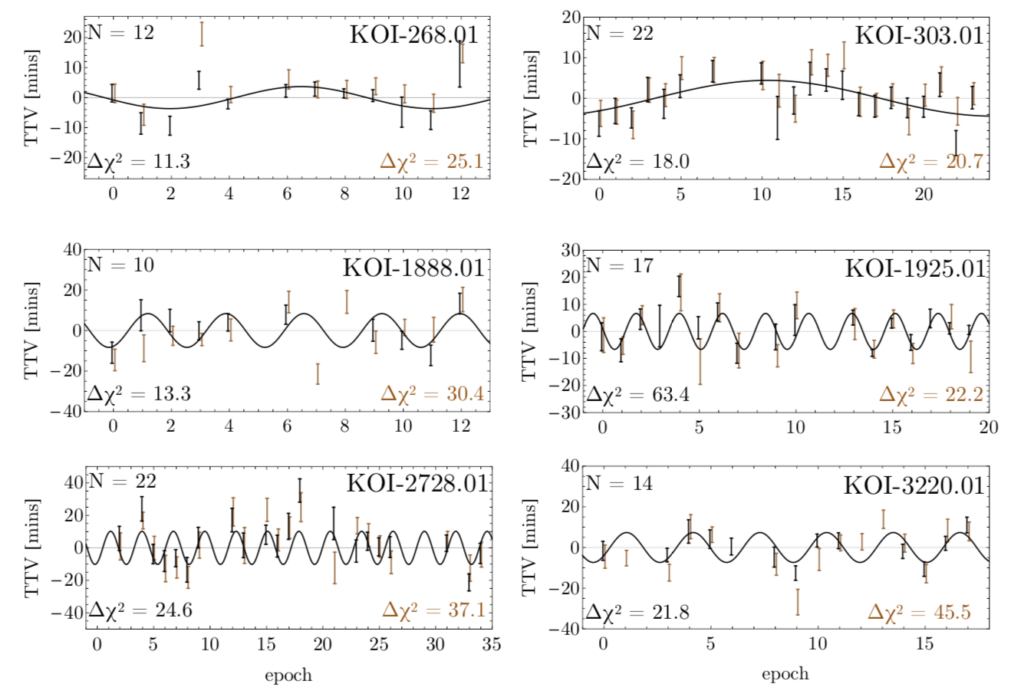
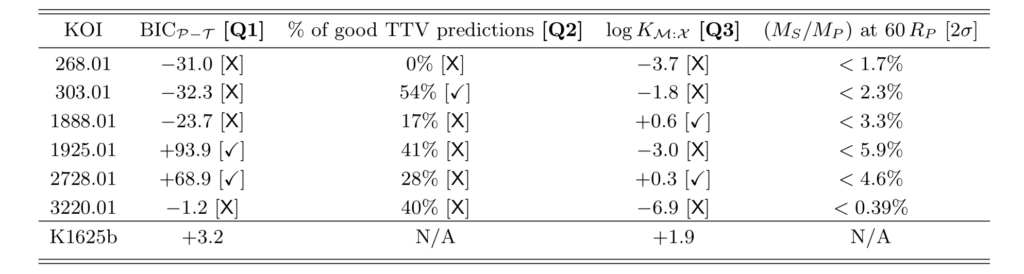
Transit Timing Variations (TTVs) is one of the methods of finding exoplanets and exomoons. Using this method, scientists try to determine whether planets are “wobbling.” Planets are expected to orbit around their stars like clocks – coming back after the same amount of time if there is nothing else affecting them gravitationally. If there is some gravitational tug acting on a planet, then it might be a moon causing the wobbling, which results in the change of the orbiting time (“orbital period”). The problem is that TTVs are quite common, and it might be another unseen planet causing the gravitational tug on the planet being observed. In Question 1, the author is interested in any evidence for TTV for the six KOIs. He found that only two of the six KOIs indicate TTVs (Table 1). However, even these two results were rather peculiar. The telescopes usually collect data by epochs – it’s like taking a photograph at evenly spaced time intervals. So, when the author analyzed the epochs of these two KOIs, he divided them into two groups, and one of the groups showed a positive result for having TTV, and the other showed the negative one.
Question 2: Is the wobbling sinusoidal?
A periodic TTV is a signal that we expect a moon to cause if it’s there. Exomoons are expected to cause sinusoidal TTVs with a period governed by the period of the moon orbiting its host planet. Question 2 tests whether the TTVs are periodic. The author used the cross-validation technique: he took 80% of the data for a training set, and 20% for a hold-out set. In this technique, scientists imagine that they have only 80% (in this case) of the data, and leave 20%, pretending that this is some newly taken data. Running the model on the training set, scientists then test the model against the hold-out set. This is done to see if the model can generalize to some independent or unknown data (the hold-out set). Usually, scientists repeat this process thousands of times, changing training and hold-out segments to minimize biases. The author of the paper changed the training and hold-out sets times. What he actually found is that only KOI-303.01 had the periodic TTV he was looking for (Table 1), but this KOI failed Question 1 above!

the χ2 test (a statistical test of how good predictions match experimental results) shown in the lower-left corner. The results in brown are the results from a different analysis. Figure 1 in the paper.Question 3: Do the observations support a statistically significant non-zero moon mass?
Question 3 tests if the exomoon model is fitted to the data, will it result in a moon with a non-zero mass? The non-zero mass moon model is a model which produces a gravitational influence as well as producing a dip in the intensity of starlight due to the moon passing in front of the star. As shown in Table 1, KOIs-268.01, 303.01, 1925.01, 3220.01 resulted in a zero-mass moon thus failing the test, and only KOIs-1888.01, 2728.01 passed the test. Moreover, some of the author’s exomoon fits converge to unphysical solutions. KOIs-268.01 & 2728.01 favored negative-radius moons, which is permitted by the author’s exomoon fits, but not by common sense!
The case of KOI-2728.01 is rather interesting because it passed 2 out of 3 tests, only missing a periodic TTV. One explanation is that the TTVs were caused by active stellar surface (starspots) just like in Kepler-17b. Nonetheless, given that KOI-2728.01 favored a negative-radius moon, the author did not conclude this KOI to be an exomoon candidate.
No happy ending?
The recent study, which the author referred his paper to, proposed six exomoon candidates, claiming they have periodic TTVs. An independent analysis by D. Kipping proposes 3 key tests for exomoon candidacy, and shows that none of the six KOIs passed these new tests. Furthermore, another recent analysis concluded that the six KOIs described in the paper could not host exomoons. If at least one of them had passed the test, it would be a major contribution to exomoon research, since there is still only one exomoon candidate (Kepler 1625b-I, claimed by the author) in the literature. When a claim as enormous as six exomoons is made, it deserves the attention of the exoplanet community. Despite the results of this paper, we know very well from studying the Solar System that planets definitely have moons – it’s just a matter of time until we’ll be able to finally observe them around exoplanets.
Astrobite edited by: Sasha Warren, Jessica May Hislop
Featured image credit: Sci-News.com.
Russian
Название: Независимый анализ шести недавно заявленных кандидатов на экзолуны
Авторы: Дэвид Киппинг
Учреждение первого автора: факультет астрономии Колумбийского университета, Нью-Йорк, штат Нью-Йорк, США.
Статус: принято в The Astrophysical Journal Letters
Спутники в нашей Солнечной системе очень распространены. Фактически, мы видим одного каждую ночь. Мы также знаем о Фобосе и Деймосе, океанических мирах Юпитера и даже о метане на самом большом из спутников Сатурна. Мысль о планете с луной настолько естественна для нас – мы наблюдали их множество в Солнечной системе. Однако, есть ли у планет в других планетных системах (мы называем их «экзопланетами») луны? Что ж, мы не знаем, наблюдали ли мы что-либо ещё. В литературе есть только одна заявленная «экзолуна» (луна планеты в планетной системе за пределами нашей). Недавнее исследование утверждает, что обнаружило шесть новых экзолун. Это большое дело! В случае подтверждения, такое открытие явится гигантским шагом вперёд в обнаружении экзолуний. В нашей Солнечной системе исследования лун смогли многое рассказать нам о формировании системы, составе планет и потенциальных подсказках того, как зародилась жизнь на Земле. Следовательно, открытие экзолун может помочь нам понять наблюдаемые нами экзопланеты. В сегодняшней статье был проведён тщательный анализ каждого из этих шести кандидатов на экзолуны и ни один и этих кандидатов не был подтверждён экзолуной.
Шесть Кеплеровских объектов интереса (КОИ) предлагаются для размещения кандидатов в экзолуны: KОИ-268.01, 303.01, 1888.01, 1925.01, 2728.01 и 3320.01. Найти экзопланету – уже непростая задача, но найти экзолуну ещё сложнее. Несмотря на большой успех поисков экзопланет, экзолуны трудно обнаружить всеми существующими методами таких поисков, поскольку они должны быть значительного размера, чтобы быть замеченными. Вот почему проводятся различные статистические анализы, чтобы найти экзолуны вокруг обнаруженных экзопланет.
Анализ в этой статье основан на трёх вопросах. По словам Дэвида Киппинга, для настоящей экзолуны ответы на все эти вопросы должны быть “да”.
Вопрос 1: Видим ли мы колебания планет?
Вариация времени прохождения (ВВП) – это один из методов поиска экзопланет и экзолун. Используя этот метод, учёные пытаются определить, «качаются» ли планеты. Ожидается, что планеты будут вращаться вокруг своих звёзд, как часы – возвращаясь через то же время, если ничто другое не влияет на них гравитационно. Если на планету действует какая-то гравитационная тяга, то это может быть луна, вызывающая колебание, что приводит к изменению времени обращения («орбитальный период»). Проблема в том, что ВВП довольно распространены, и это может быть другая невидимая планета, вызывающая гравитационное притяжение наблюдаемой планеты. В Вопросе 1 автора интересуют любые доказательства существования ВВП для шести КОИ. Он обнаружил, что только два из шести КОИ указывают на ВВП (Таблица 1). Однако, даже эти два результата были довольно своеобразными. Телескопы обычно собирают данные по эпохам – это всё равно, что делать снимок через равные промежутки времени. Итак, когда автор анализировал эпохи этих двух КОИ, он разделил их на две группы, одна из которых показала положительный результат на наличие ВВП, а другая – отрицательный.
Вопрос 2: является ли колебание синусоидальным?
Периодическая ВВП – это сигнал, который, как мы ожидаем, вызовет луна, если она там есть. Ожидается, что экзолуны вызовут синусоидальные ВВП с периодом, определяемым периодом движения луны по орбите своей планеты-хозяина. Вопрос 2 проверяет, являются ли ВВП периодическими. Автор использовал метод перекрестной проверки: он взял 80% данных для обучающей выборки и 20% для выборке удержания. В этом методе учёные воображают, что у них есть только 80% (в данном случае) данных, и оставляют 20%, делая вид, что это новые полученные данные. Запуская модель на обучающей выборке, учёные затем проверяют модель на удерживающей выборке. Это делается для того, чтобы увидеть, можно ли обобщить модель на некоторые независимые или неизвестные данные (удерживаемая выборка). Обычно учёные повторяют этот процесс тысячи раз, изменяя сегменты тренировки и удержания, чтобы минимизировать ошибки. Автор статьи раза менял тренировочную и удерживающую выборки. На самом деле. он обнаружил, что только у KОИ-303.01 был периодическая ВВП, которую он искал (Таблица 1), но этот KОИ не прошёл Вопрос 1 выше!
Вопрос 3: подтверждают ли наблюдения статистически значимую массу Луны, отличную от нуля?
Вопрос 3 проверяет, если данные экзолуны соотнести к модели, приведёт ли она к появлению луны с ненулевой массой? Модель луны с ненулевой массой – это модель, которая создаёт гравитационное влияние, а также вызывает падение интенсивности звёздного света из-за того, что Луна проходит перед звездой. Как показано в Таблице 1, КОИ-268.01, 303.01, 1925.01, 3220.01 привели к получению луны нулевой массы, таким образом, не прошли испытание, и только KОИ-1888.01, 2728.01 прошли испытание. Более того, некоторые из авторских моделей экзолун сходятся к нефизическим решениям. KОИ-268.01 и 2728.01 соответствуют спутникам с отрицательным радиусом, что разрешено моделью автора, но не здравым смыслом!
Случай КОИ-2728.01 весьма интересен тем, что он прошёл 2 из 3 тестов, пропустив лишь периодическую ВВП. Одно из объяснений состоит в том, что ВВП были вызваны активной звёздной поверхностью (звёздными пятнами), как и в Kepler-17b. Тем не менее, учитывая, что KОИ-2728.01 одобрял луну с отрицательным радиусом, автор не пришёл к выводу, что этот КОИ является кандидатом на экзолуну.
Нет счастливого конца?
В недавнем исследовании, на которое автор ссылался в своей статье, было предложено шесть кандидатов на экзолуны, утверждая, что у них есть периодические ВВП. Независимый анализ, проведенный Д. Киппингом, предлагает 3 ключевых теста на кандидатуру экзолуны и показывает, что ни один из шести KОИ не прошёл эти новые тесты. Более того, другой недавний анализ пришёл к выводу, что шесть КОИ, описанные в статье, не могут содержать экзолуны. Если бы хотя бы один из них прошёл тест, это было бы важным вкладом в исследования экзолун, поскольку в литературе всё ещё есть только один кандидат на экзолуну (Kepler 1625b-I, как утверждает автор). Когда делается такое большое заявление, как шесть экзолун, оно заслуживает внимания сообщества экзопланет. Несмотря на результаты этой статьи, из изучения Солнечной системы мы очень хорошо знаем, что у планет определенно есть луны – это всего лишь вопрос времени, когда мы наконец сможем наблюдать за ними вокруг экзопланет.
Астробайт отредактирован Сашей Уоррен и Джессикой Мэй Хислоп
Изображение взято с: Sci-News.com.