Title: Neutron star mass estimates from gamma-ray eclipses in spider millisecond pulsar binaries
Authors: C. J. Clark, M. Kerr, E. D. Barr, et. al (26 additional authors)
First Author’s Institution: Max Planck Institute for Gravitational Physics (Albert Einstein Institute)
Status: Published in Nature Astronomy [open access]
What’s it like inside a neutron star? We certainly know it’s cozy– these supernova remnants are so dense that a teaspoon of neutron star (NS) would weigh as much as Mount Everest! However, beyond the basics, there are many unanswered questions about the internal structure and composition of neutron stars. In particular, we have not yet calculated the equation of state (EoS) of NS matter, which describes the relationship between pressure and energy density throughout the NS. This is a technical way of saying that we have very little information about the structure and properties of the matter inside NSs. Most neutron stars are pulsars, rapidly rotating NSs with strong magnetic fields. The emission from these pulsars presents us a unique opportunity to study nuclear physics at its most extreme.
Today’s authors use the Fermi Gamma-Ray Space Telescope to study pulsars in “spider” binary systems: pulsars in a binary orbit with a typical star. Spider systems are classified into “black widow” systems (with stellar companions lighter than 0.1 solar masses) and “redback” systems (heavier companions). Like the actual spiders for which they are named, the pulsars in these systems tear apart their companion with their extremely high-energy winds, causing the outer layers of the companion star to be ripped violently away from its surface through a process called ablation. These systems can be studied all across the electromagnetic spectrum: there is pulsed radio emission from the rotating pulsar, optical emission from the stellar companion, and high-energy particles arising from the pulsar wind and the shock it creates when it runs into the dense gas in the system.
How Can We Weigh Pulsars?
With so many different avenues to explore when studying these systems, you may be surprised to learn that cutting-edge studies like today’s focus on measuring something as simple as the pulsar’s mass! Accurate measurements of NS masses are crucial for determining the exact equation of state inside– each theory for the EoS has a theoretical maximum NS mass, so finding a NS which is heavier can rule out that theory (or, finding a lack of sufficiently heavy neutron stars can help rule out another). Astronomers typically estimate the pulsar mass by looking at the optical light curve, or how the optical brightness changes across the orbit– but these estimates make several assumptions, and depend heavily on the inclination angle i of the system relative to us.
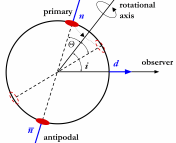
Imagine holding a frisbee or dinner plate at arm’s length: if you’re looking at it face-on, you can see the entire outer perimeter of the disk all at once, and the inclination angle is low. Conversely, if you angle the plate so you only see a thin edge, you’re looking at it edge-on, and the inclination angle is high (up to 90 degrees). In face-on binaries, we can see both the pulsar and companion pretty much constantly; in edge-on binaries, there are points where one object will move directly in front of the other, blocking our line of sight to the object in back– the exact same circumstances which lead to solar and lunar eclipses on Earth. When we try to measure the masses of pulsars in binaries, the estimated pulsar mass depends heavily on the binary’s inclination angle i (1/sin^3(i) for the math-minded audience) – so the mass measurement problem becomes an inclination angle measurement problem, but measuring those using optical light curves can be inaccurate. Estimating the inclination angle this way requires a laundry list of assumptions about the companion star, which can introduce many systematic biases into the final result.
Total Eclipse of the Pulsar
Today’s authors use a new method to constrain the possible inclination angles, and thus the masses, of several spider binary systems. The authors use observations of 47 gamma-ray emitting spider systems over an 11-year time frame to search for gamma-ray eclipses: points in the orbit where the gamma-ray emission disappears. Since the gamma-rays come from the pulsar, eclipses can only happen when the companion moves directly in between us and the pulsar, meaning the system must be very close to edge-on, placing lower limits on the inclination angle of the system, and thus strict limits on the pulsar mass.
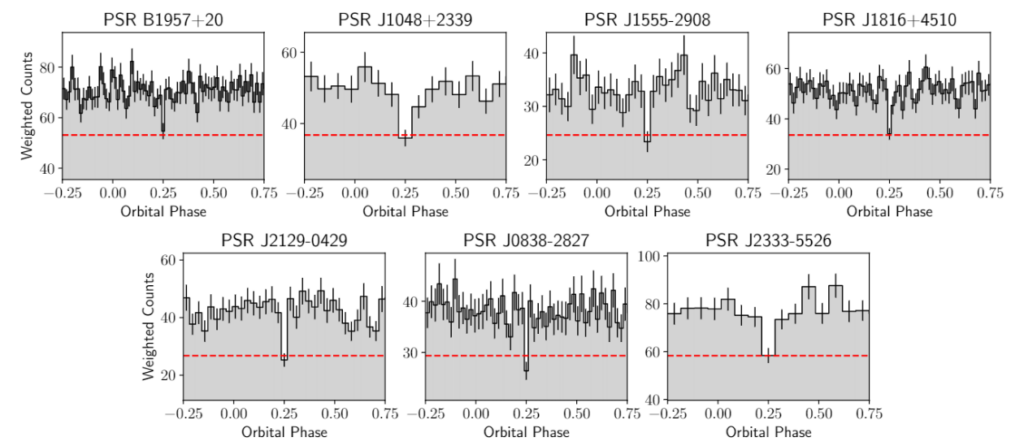
Only seven of the binary systems studied showed measurable gamma-ray eclipses; figure 1 shows the gamma-ray orbital light curves for each of them. Each system shows a pronounced dip in the number of detected gamma-ray photons at an orbital phase of 0.25, which by convention is the point in the orbit when the companion is directly in front of the pulsar. Since gamma-rays are so rare and energetic, the number of detected gamma-ray photons is used as a proxy for the flux. Notice how rare gamma-rays are: the most significant eclipse occurs in PSR J2129-0429, which shows a deficit of about 20 gamma-ray photons at the eclipse- remember that this is over the span of 11 years of data!
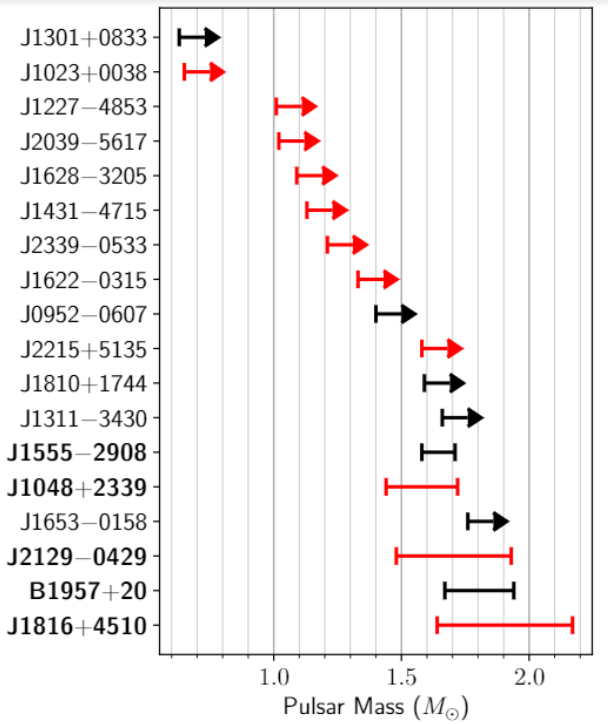
All of these spider systems have been intensely studied for many years, meaning we know their orbital parameters and the properties of the companion stars quite well. The authors used this information to create incredibly detailed simulations of each binary system. By simulating them over a range of test inclination angles, and comparing the resulting simulated gamma-ray eclipses to what is actually seen in reality, they were able to calculate limits on each system’s inclination angle with unprecedented precision. These inclination angle ranges lead to the most up-to-date constraints on the pulsar masses in each system, presented in Figure 2.
As a final demonstration of the power of this method, the black widow pulsar B1957+20 (second from the bottom in Figure 2) had a previously estimated mass of ~2.4 solar masses, much heavier than any other known neutron star and heavier than most proposed equations of state would allow. However, this estimate used the optical light curve modeling technique, and led to an estimated inclination angle of ~63-67 degrees; the gamma-ray eclipses found here suggest an inclination angle of at least 84 degrees, leading to a much more reasonable pulsar mass estimate, and salvaging many promising EoS theories in the process!
Astrobite edited by Ben Cassese
Featured image credit: Dana Berry/NASA Goddard Space Flight Center



This is a very nice summary — particularly the part putting the individual photon counts in perspective; it’s amazing what those instruments can do and what clever humans can statistically extract from them — of a very nice paper. Thank you!